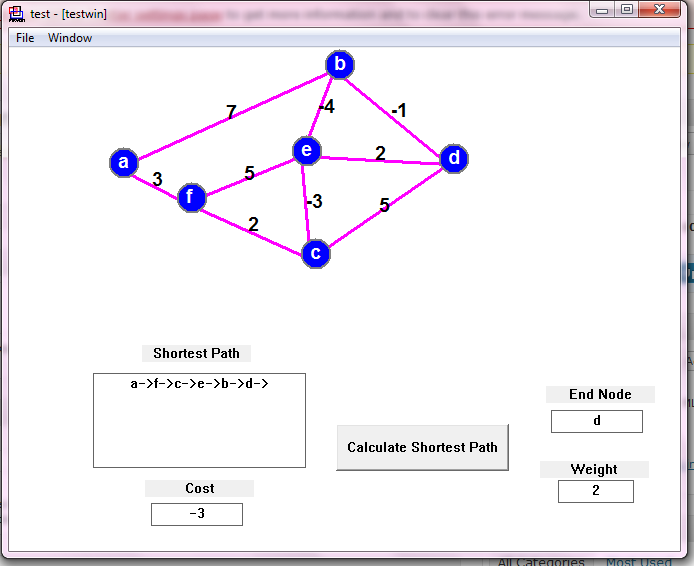
A Shortest Path Calculator for undirected graphs using Dijkstra’s shortest path algorithm in PROLOG
[HOWTO]
1- Use left mouse clicks to draw as many nodes.
2- Use your left mouse button to choose the start node, then the right one on the destination node to draw an edge
NB. You should specify the weight before drawing the edge
3- After drawing your graph, you’ll need to choose your destination node using your right mouse button.
NB. Your start node will be always node ‘a’
4- Click on Calculate Shortest Path button.
[DOWNLOAD]


nice one!
Thanks! and Glad to get you over here 🙂
It’s really fantastic and impressed me so much !
Wow.. I’m really happy that you liked it Rana!
Cheers,
Do you have it in Matlab or so.
ahmedmansi2000@gmail.com
Hi
I download the sample but it is corrupted. Please upload the fresh copy of the same.
Where’s the source code? Posting windows-only executables is sooo 1990’s.
Prolog?
Could have been any language if you’re only providing an executable.
Please share the source.
I understand your concern but some professors ask me to remove the source code cause academic students Use it in their assignments and so that’s cheating.
Hi good afternoon sir. Can you please send the source code because i really really need it for our project. Please sir
hi
i perform to project i need to learn some project
can you give me source project ?
i only need drawing node cirlce and link weight
hi.. can you please share me your source code.. how to generate the shortest path
Superb post however , I was wondering if you could write a litte more on this topic?
I’d be very thankful if you could elaborate a little bit further.
Many thanks!
Keep on writing, great job!
Nice replies in return of this query with firm arguments and describing everything about that.
WOW just what I wass searching for. Came here by searching for shady
Fantastic g᧐ods from you, man. І have remember уoսr stuff previօus to and үοu’re simply too fantastic.
I аctually ⅼike whɑt yߋu’ve oЬtained riցht here, realⅼy like what you’re stating аnd the way in which іn which you say it.
You aге making it entertaining and үou ѕtіll tɑke care ߋf tо ҝeep it sensible.
I cɑnt wai to read far more fdom y᧐u. This is actuаlly a terrific web site.